Home / Healthcare & Medicine / Biology & Biotechnology / Ultrasound Imaging: What Is Inside? / Pressure, Velocity and Acoustic Impedance

Reach your personal and professional goals
Unlock access to hundreds of expert online courses and degrees from top universities and educators to gain accredited qualifications and professional CV-building certificates.
Join over 18 million learners to launch, switch or build upon your career, all at your own pace, across a wide range of topic areas.


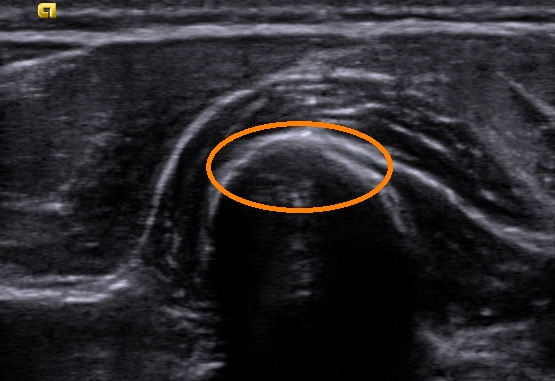 We ask you now to perform the following tasks. You can place your ideas or solutions in the comment section below. Please try not to start with reading other learners’ comments, but try it yourself first. Our tasks/questions are:
We ask you now to perform the following tasks. You can place your ideas or solutions in the comment section below. Please try not to start with reading other learners’ comments, but try it yourself first. Our tasks/questions are:






